|
ACTA
MATHEMATICA
|
p. 265 - 284
Slowly varying solutions of a class of first order systems
of nonlinear differential equations
J. Jaroš and Takaŝi Kusano
Received: November 12, 2012; Accepted: February 6, 2013
Abstract. We analyze positive solutions of the two-dimensional systems of nonlinear differential equations
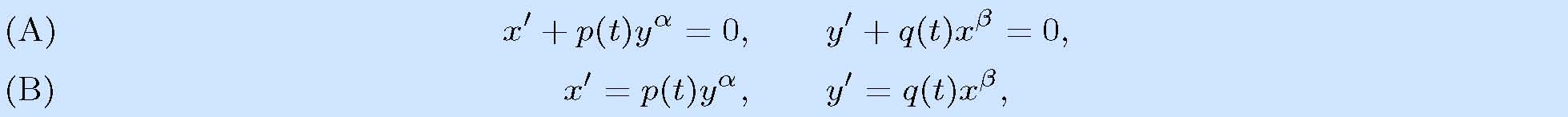
in the framework of regular variation and indicate the situation in which system (A) (resp. (B) possesses decaying solutions (resp. growing solutions) with precise asymptotic behavior as $t \to \infty$.
Keywords: systems of differential equations; positive solutions; asymptotic behavior; regularly varying functions.
AMS Subject classification: Primary: 34C11, 26A12
PDF Compressed Postscript Version to read
ISSN 0862-9544 (Printed edition)
Faculty of Mathematics, Physics and Informatics
Comenius University
842 48 Bratislava, Slovak Republic
Telephone: + 421-2-60295111 Fax: + 421-2-65425882
e-Mail: amuc@fmph.uniba.sk Internet: www.iam.fmph.uniba.sk/amuc
© 2013, ACTA MATHEMATICA UNIVERSITATIS COMENIANAE