| |
|
|
| |
Volume 1, Issue 2, Article 14 |
|
|
|
|
|
| |
Regularity results for vector fields of bounded distortion and applications
|
| |
|
Authors: |
Alberto Fiorenza, Flavia Giannetti, |
|
| |
|
Keywords:
|
Reverse Inequalities, Finite Distortion Vector Fields, Div-Curl Vector Fields, Elliptic Partial Differential Equations |
|
| |
|
Date Received:
|
17/01/00 |
|
| |
|
Date Accepted:
|
04/04/00 |
|
| |
|
Subject Codes: |
35J60,26D15.
|
|
| |
|
Editors: |
Saburou Saitoh, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
In this paper we prove higher integrability results for vector fields 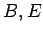 , , 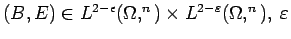 small, such that small, such that 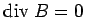 , , 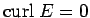 satisfying a ``reverse'' inequality of the type satisfying a ``reverse'' inequality of the type with 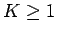 and and 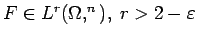 . Applications to the theory of quasiconformal mappings and partial differential equations are given. In particular, we prove regularity results for very weak solutions of equations of the type . Applications to the theory of quasiconformal mappings and partial differential equations are given. In particular, we prove regularity results for very weak solutions of equations of the type
If  , in the homogeneous case, our method provides a new proof of the regularity result where , in the homogeneous case, our method provides a new proof of the regularity result where 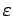 is sufficiently small. A result of higher integrability for functions verifying a reverse integral inequality is used, and its optimality is proved. is sufficiently small. A result of higher integrability for functions verifying a reverse integral inequality is used, and its optimality is proved.
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|



