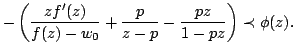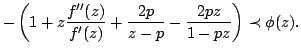JIPAM

Coefficient Bounds for Meromorphic Starlike and Convex Functions  
 
  Authors: See Keong Lee, V. Ravichandran, Supramaniam Shamani,  
  Keywords: Univalent meromorphic functions; starlike function, convex function, Fekete-Szegö inequality.  
  Date Received: 30/01/2008  
  Date Accepted: 03/05/2009  
  Subject Codes:

Primary 30C45, Secondary 30C80.

  
  Editors: Sever S. Dragomir,  
 
  Abstract:

In this paper, some subclasses of meromorphic univalent functions in the unit disk are extended. Let denote the class of normalized univalent meromorphic functions $ f$ in with a simple pole at . Let be a function with positive real part on with $ phi(0)=1$, which maps onto a region starlike with respect to which is symmetric with respect to the real axis. The class consists of functions $ fin U(p)$ satisfying

   
The class $ sum(p, phi)$ consists of functions $ fin U(p)$ satisfying
   
The bounds for $ w_0$ and some initial coefficients of in and are obtained. ;


This article was printed from JIPAM
http://jipam.vu.edu.au

The URL for this article is:
http://jipam.vu.edu.au/article.php?sid=1127