| |
|
Abstract: |
In this paper, we study the Baker's superstability for the following functional equation where 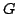 is a locally compact group, is a locally compact group, 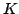 is a compact subgroup of is a compact subgroup of 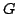 , , 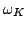 is the normalized Haar measure of is the normalized Haar measure of 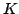 , , 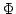 is a finite group of is a finite group of 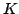 -invariant morphisms of -invariant morphisms of 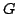 and and 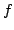 is a continuous complex-valued function on is a continuous complex-valued function on 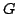 satisfying the Kannappan type condition, for all satisfying the Kannappan type condition, for all 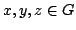 | 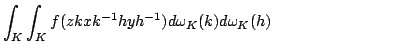
| (*) |
|



