| |
|
|
| |
Volume 6, Issue 2, Article 44 |
|
|
|
|
|
| |
A Sawyer Duality Principle for Radially Monotone Functions in $R^n$.
|
| |
|
Authors: |
Sorina Barza, Maria Johansson, Lars-Erik Persson, |
|
| |
|
Keywords:
|
Duality theorems, Radially monotone functions, Weighted inequalities. |
|
| |
|
Date Received:
|
09/02/05 |
|
| |
|
Date Accepted:
|
14/02/05 |
|
| |
|
Subject Codes: |
Prim. 26D15, 47B38; Sec. 26B99, 46E30.
|
|
| |
|
Editors: |
Constantin P. Niculescu, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
Let 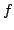 be a non-negative function on be a non-negative function on 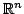 , which is radially monotone , which is radially monotone 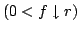 . For . For 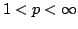 , , 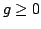 and and 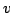 a weight function, an equivalent expression for a weight function, an equivalent expression for is proved as a generalization of the usual Sawyer duality principle. Some applications involving boundedness of certain integral operators are also given.
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|



