| |
|
|
| |
Volume 6, Issue 2, Article 54 |
|
|
|
|
|
| |
Strongly Nonlinear Elliptic Unilateral Problems in Orlicz Space and $L^1$ Data
|
| |
|
Authors: |
L. Aharouch, M. Rhoudaf, |
|
| |
|
Keywords:
|
Orlicz Sobolev spaces, Boundary value problems, Truncations, Unilateral problems. |
|
| |
|
Date Received:
|
21/12/04 |
|
| |
|
Date Accepted:
|
06/04/05 |
|
| |
|
Subject Codes: |
35J60
|
|
| |
|
Editors: |
Alberto Fiorenza, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
In this paper, we shall be concerned with the existence result of Unilateral problem associated to the equations of the form, where 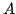 is a Leray-Lions operator from its domain is a Leray-Lions operator from its domain 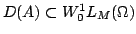 into into 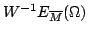 . On the nonlinear lower order term . On the nonlinear lower order term 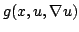 , we assume that it is a Carathéodory function having natural growth with respect to , we assume that it is a Carathéodory function having natural growth with respect to 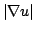 , and satisfies the sign condition. The right hand side , and satisfies the sign condition. The right hand side 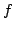 belongs to belongs to 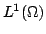 . .
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|



