 |
Journal of Integer Sequences, Vol. 13 (2010), Article 10.4.8 |
 |
Journal of Integer Sequences, Vol. 13 (2010), Article 10.4.8 |
Abstract:
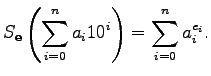
(Concerned with sequence
A007770.)
Received April 7 2010; revised version received April 12 2010. Published in Journal of Integer Sequences, April 15 2010.