[Ai]     C. Aistleitner, Quantitative uniform distribution results for geometric progressions. Israel J. Math., 204, 1: 155–197, 2014. MR3273455

[AB1]     C. Aistleitner and I. Berkes, On the central limit theorem for f(n_kx). Probab. Theory Related Fields, 146, 1–2:267–289, 2010. MR2550364

[AB2]     C. Aistleitner and I. Berkes, Probability and metric discrepancy theory. Stoch. Dyn., 11, 183–207, 2011. MR2771348

[AB3]     C. Aistleitner and I. Berkes, Limit distributions in metric discrepancy theory. Monatsh. Math., 169, 3–4: 253—265, 2013. MR3019283

[ABT]     C. Aistleitner, I. Berkes, and R.F. Tichy, Lacunary sequences and permutations. Dependence in probability, analysis and number theory. A volume in memory of Walter Philipp, Kendrick Press. 35–49, 2010. MR2731043

[Bu1]     K. Burdzy, The Search for Certainty. World Scientific, 2009. MR2510150

[Bu2]     K. Burdzy, Resonance: From Probability to Epistemology and Back. Imperial College Press, 2016. MR3468703

[Du]     R. Durrett, Probability: theory and examples, 3rd edition. Duxbury advanced series, 2005. MR1609153

[CDO]     S. Chen, J. Dick, and A.B. Owen, Consistency of Markov chain quasi-Monte Carlo on continuous state spaces. Ann. Statist., 39, 2:673–701, 2011. MR2816335

[CMNO]     S. Chen, M. Matsumoto, T. Nishimura, and A.B. Owen, New inputs and methods for Markov chain quasi-Monte Carlo. In Monte Carlo and quasi-Monte Carlo methods 2010. Springer Proc. Math. Stat.  23, 313–327, Springer, 2012. MR3173841

[DELV]     H. Davenport, P. Erd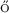s, and W.J. LeVeque, On Weyl’s criterion for uniform distribution. Michigan Math. J., 10, 3:311–314, 1963. MR0153656

[DT]     M. Drmota and R.F. Tichy, Sequences, Discrepancies, and Applications. Lecture Notes in Mathematics 1651, Springer, Berlin, 1997. MR1470456

[DTW]     M. Drmota, R.F Tichy, and R. Winkler, Completely uniformly distributed sequences of matrices. In: Number-Theoretic Analysis. Lecture Notes in Math. 1452, pp. 43–57, Springer, 1990. MR1084637

[EK]     P. Erd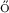s and M. Kac, The Gaussian law of errors in the theory of additive number theoretic functions. Amer. J. Math., 62, 1:738–742, 1940. MR0002374

[Fr]     J.N. Franklin, Deterministic simulation of random processes, Math. Comp., 17, 28–59, 1963. MR0149640

[Go]     M. Goldstern, Eine Klasse volständig gleichverteiler Folgen. In Zahlentheoretishe Analysis, II Lecture Notes in Math. 1262, pp. 37–45, Springer, 1987. MR1012958

[Ho1]     P.J. Holewijn, Note on Weyl’s criterion and the uniform distribution of independent random variables. Ann. Math. Stat. 40, 1124–1125, 1969. MR0240855

[Ho2]     P.J. Holewijn, On the Uniform Distribution of Sequences of Random Variables. Z. Wahrscheinlichkeitstheorie verw. Geb., 14, 89–92, 1969. MR0264735

[Ka1]     M. Kac, Statistical Independence in Probability, Analysis and Number Theory. Mathematical Association of America, 1959. MR0110114

[Ka2]     M. Kac, Probability methods in some problems of analysis and number theory. Bull. Amer. Math. Soc., 55, 641–665, 1949. MR0031504

[Kem]     J.H.B. Kemperman, Probability methods in the theory of distributions modulo one. Compositio Mathematica, 16 106–137, 1964. MR0173281

[Ke1]     H. Kesten, Uniform Distribution mod 1. Ann. Math.,71, 2:445–471, 1960. MR0113864

[Ke2]     H. Kesten, Uniform Distribution mod 1 (II). Acta Arith., 7, 4:355–380, 1962. MR0142532

[Kh]     D. Khoshnevisan, Normal numbers are normal. Clay Mathematics Institute Annual Report, 15, continued pp. 27—31, 2006.

[Kn1]     D.E. Knuth, Construction of a random sequence. BIT Numerical Mathematics, 5, 4:246–250, 1965. MR0197434

[Kn2]     D.E. Knuth, The Art of Computer Programming, volume 2/Seminumerical Algorithms, 3rd edition. Addison-Wesley, 1997. MR3077152

[Kol1]     A.N. Kolmogorov, Grundbegriffe der Wahrscheinlichkeitsrechnung, Ergebnisse der Mathematik und ihrer Grenzgebeite. J. Springer, 1933. MR0362415

[Kol2]     A.N. Kolmogorov, Tri podhoda k opredeleniju ponjatija “količevstvo informacii” (Three approaches to the definition of the concept “quantity of information”.). Problemy peredači informacii 1, 3–11, 1965.

[Kok]     J.F. Koksma, Ein mengentheoretischer Satz über die Gleichverteilung modulo Eins. Compositio Math., 2, 250–258, 1935. MR1556918

[Kr1]     N.M. Korobov, On functions with uniformly distributed fractional parts (Russian). Doklady Akad. Nauk SSSR (N.S.), 62, 21–22, 1948. MR0027012

[Kr2]     N.M. Korobov, Some problems on the distribution of fractional parts (Russian). Uspehi Matem. Nauk SSSR (N.S.), 4, 1(29):189–190, 1949. MR0031948

[Kr3]     N.M. Korobov, Concerning some questions of uniform distribution (Russian). Izvestiya Akad. Nauk SSSR. Ser. Mat.  14, 215–238, 1950. MR0037876

[Kr4]     N.M. Korobov, Bounds of trigonometric sums involving completely uniformly distributed functions (Russian). Soviet Math. Dokl., 1, 923–926, 1960. MR0130527

[Kr5]     N.M. Korobov, Exponential Sums and their Applications. Mathematics and Its Applications, 80, Kluwer, 1992. MR1162539

[KN]     L. Kuipers and H. Niederreiter, Uniform Distribution of Sequences. Wiley and Sons, 1974. MR0419394

[Lac]     M.B. Lacaze, Estimation de moyennes et fonctions de répartition de suites d’échantillonnage. Ann. Inst. Henri Poincaré Probab. Stat., 9, 2:145–165, 1973. MR0356199

[Lam]     M. van Lambalgen, Randomness and Foundations of Probability: von Mises’ axiomatisation of random sequences. Statistics, probability and game theory: Papers in honor of David Blackwell, 347–367, IMS, 1996. MR1481789

[Le1]     L. Levin, On the notion of a random sequence. Soviet Math. Dok. 14 1413–1416, 1973.

[Le2]     M.B. Levin, Discrepancy Estimates of Completely Uniformly Distributed and Pseudorandom Number Sequences. Internat. Math. Res. Notices 22, 1231–1251, 1999. MR1731474

[Li]     N. Limić, On completely equidistributed numbers. Math. Commun. 7, 103–111, 2002. MR1952752

[Lo]     R.M. Loynes, Some results in the probabilistic theory of asymptotic uniform distribution modulo 1. Z. Wahrscheinlichkeitstheorie verw. Geb. 26, 33–41, 1973. MR0329051

[Ly]     R. Lyons, Strong laws of large numbers for weakly correlated random variables. Michigan Math. J., 35, 3:353–359, 1988. MR0978305

[ML]     P. Martin-Löf, The definition of random sequences. Inform. Control 9, 602–619, 1966. MR0223179

[MF]     M. Mendès France, Nombres normaux. Applications aux fonctions pseudo-aléatoires. J. Anal. Math., 20, 1:1–56, 1967. MR0220683

[Ms]     R. von Mises, Probability, Statistics and Truth, 2nd revised English edn., Dover, New York, 1957. MR0090158

[NT1]     H. Niederreiter and R.F. Tichy, Solution of a problem of Knuth on complete uniform distribution of sequences. Mathematika, 32, 26–32, 1985. MR0817103

[NT2]     H. Niederreiter and R.F. Tichy, Metric theorems on uniform distribution and approximation theory. Journées arithmétiques de Besançon (Besançon, 1985). Astérisque 147–148, 319–323, 1987. MR0891439

[OT]     A.B. Owen and S.D. Tribble, A quasi-Monte Carlo Metropolis algorithm. Proc. Natl. Acad. Sci. USA, 102, 25:8844–8849, 2005. MR2168266

[SZ]     R. Salem and A. Zygmund, On Lacunary Trigonometric Series. Proc. Natl. Acad. Sci. USA, 33, 11:333–338, 1947. MR0022263

[SP]     O. Strauch and S. Porubský, Distribution of Sequences: A Sampler. Peter Lang, 2005. MR2290224

[Ti]     R.F. Tichy, Ein metrischer Satz über vollständig gleichverteile Folgen. Acta Arith. 48, 2:197–207, 1987. MR0895440

[TO]     S.D. Tribble and A.B. Owen, Construction of weakly CUD sequences for MCMC sampling. Electron. J. Stat., 2, 634–660, 2008. MR2426105

[We]     H. Weyl, Über die Gleichverteilung von Zahlen mod. Eins. Math. Ann., 77, 313–352, 1916. MR1511862