Chapter 3.2

  Similarity Symmetry Rosettes
  and Ornamental Art

The simplest of the symmetry groups of the type C_nK (nK) is the symmetry group K (n = 1) generated by a central dilatation with the dilatation coefficient k. In this transformation, for every two original points A, B and their images A' = K(A), B' = K(B), the vector relationship (A',B') = k(A,B) holds. In this way, all the lines of the image are parallel to the homologous lines of the original figure (for k > 0) or antiparallel, i.e. parallel and oppositely oriented (for k < 0). Hence, the form and all the angles of a figure remain unchanged under the action of the dilatation K, so the equiformity and equiangularity are the properties of a dilatation. There is also an important metric property of a dilatation: the series of the distances of successive homologous points of the dilatation K from the dilatation center, is a geometric progression with the coefficient |k|. Since all the similarity transformations contain a dilatational component, the metric property holds for all the similarity transformations. A fundamental region of the similarity symmetry group K is a part of the plane, bounded by two homologous lines of the dilatation K. The non-metric construction of similarity symmetry rosettes with the symmetry group K and their use in ornamental art, is based on the maintenance of the parallelism, without using the metric property of the dilatation K. The best way of achieving this is the use of linked successive homologous asymmetric figures of the dilatation K (for k > 0), maintaining the parallelism between homologous lines of the dilatation K (Figure 3.3).

This construction method was proposed by A.V. Shubnikov (1960). It can only be used respecting the restriction k > 0, accepted by H. Weyl (1952) and A.V. Shubnikov. For k < 0, the dilatation K = K(k), coinciding to the dilative rotation L( |k|,p), is a composite transformation consisting of the dilatation K(|k|) and the half-turn T, with the same invariant point. By accepting the restriction k > 0, symmetry groups of the type C_nK (nK) with n - an odd natural number and k < 0, are included in the type C_nL (nL), according to the relationship: C_nK = C_nL (|k|,p) = C_nL_2n(|k|,p/n) = C_nL_2n. In particular, if n = 1 and k < 0, the relationship K= L(|k|,p) holds. The simplest way to construct a figure with the symmetry group K (for k < 0), without using the metric property of the generating dilatation K is, most probably, to construct a series of homologous asymmetric figures by the dilatation K(|k|), and afterward, to copy every second figure by the half-turn T. This construction is the same as the construction of a figure with the symmetry group L(|k|,p). Owing to the complexity of the construction itself, in the earlier phases of ornamental art, it is very difficult to find examples of the consequent use of the similarity symmetry group K (for k < 0). The symmetry group K (for k > 0) occurs in ornamental art, though not frequently, due to its low degree of symmetry. It plays a special role in fine art works using the central perspective.

Figures with the symmetry group K possess a high degree of visual dynamism, producing the visual impression of centrifugal motion. They occur as enantiomorphic modifications. Polar radial rays exist. In the geometric sense, a radial ray is any half-line with the starting point in the center of the dilatation K. In the visual sense, this is a basic half-line of any series of homologous asymmetric figures of the dilatation K (Figure 3.3).

The characteristic visual properties of the symmetry group K are preserved in all the symmetry groups of the type C_nK (nK).

Every symmetry group of the type C_nK (nK) is the direct product of the symmetry groups K and C_n (n). Since the generating symmetry groups K and C_n (n) occur relatively seldom in ornamental art, the same holds for all the symmetry groups of the type C_nK (nK). A fundamental region of the symmetry group C_nK (nK) is the section of the fundamental regions of the symmetry groups K and C_n (n) with the same invariant point. Polar radial rays exist. Similarity symmetry rosettes with a symmetry group of the type C_nK (nK) may be obtained from rosettes with the symmetry group C_n (n), multiplying them by the dilatation K with the same center. The same result can be obtained multiplying by means of the n-fold rotation, a figure with the symmetry group K, belonging to a fundamental region of the symmetry group C_n (n) with the same rotation and dilatation center. Besides the construction mentioned, based on the maintenance of the parallelism, applicable when successive homologous asymmetric figures of the dilatation K (for k > 0) are linked to each other, constructions based on the metric property of the dilatation are also possible, and also the combinations of these two methods.

Owing to its complexity, the metric construction of similarity symmetry rosettes with the symmetry group C_nK (nK) was very seldom used in ornamental art. Where such attempts exist, they are usually followed by deviations from the regularity, so that similarity symmetry rosettes obtained only suggest the symmetry C_nK (nK), without satisfying it in the strict sense. Aiming for maximal constructional simplicity, usually triumphant is the simplest as possible metric regularity, where the geometric progression formed by distances of successive homologous points of the dilatation K from the dilatation center, is replaced by an arithmetic progression. That disturbs the parallelism, equiformity and equiangularity, and consequently, the similarity symmetry.

The above discussion refers to the case of k > 0. For k < 0 the construction itself is more complicated, despite the fact that the parallelism (antiparallelism) may be used. Then, the linking of successive homologous asymmetric figures of a dilatation, or the metric construction method cannot be exclusively used, so that, for n - an odd natural number and k < 0, adequate examples of similarity symmetry rosettes with the symmetry group C_nK (nK) are rarely found in early ornamental art. For n - a fixed even natural number, there is no difference between symmetry groups of the type C_nK (nK), depending on the sign of the dilatation coefficient k, so the relationship C_nK(k)=C_nK(-k) holds. That is because, for n - an even natural number, the half-turn already exists in the symmetry group C_n ( n), so it is included in the symmetry group C_nK ( nK) derived from it. The difference between the symmetry groups C_nK(k) and C_nK(-k) occurs only for n - a fixed odd natural number. In the symmetry group with k < 0 and n - an odd natural number, a half-turn does not exist as an independent symmetry transformation of the symmetry group C_nK (nK), but only as a part of the composite transformation K = TK(|k|). For n - an odd natural number and k < 0, the relationship C_nK= C_nL(|k|,p/n) = C_nL_2n holds.

Apart from by the construction methods mentioned above, similarity symmetry rosettes with the symmetry group C_nK (nK) may be constructed by a desymmetrization of the symmetry group D_nK ( nmK), where by a coloring or some other desymmetrization procedure, reflections are eliminated. The similarity symmetry rosettes derived often belong to similarity antisymmetry rosettes with the antisymmetry group D_nK/C_nK (nmK/nK), treated by the classical theory of symmetry as similarity symmetry rosettes with the symmetry group C_nK (nK).

The causes of the origin of similarity symmetry groups of the type C_nK (nK) in ornamental art, can be found in their visual-symbolic meaning, where two dynamic components - the centrifugal component produced by the dilatation K, and the suggestion of a rotational motion produced by the subgroup C_n (n) - come to their full expression.

Every similarity symmetry group of the type D_nK (nmK) is the direct product of the symmetry groups D_n (nm) and K. Similarity symmetry rosettes with the symmetry group D_nK ( nmK) can be constructed multiplying by the dilatation K a figure with the symmetry group D_n (nm), belonging to a fundamental region of the symmetry group K, or multiplying by the symmetries of the group D_n (nm) a figure with the symmetry group K, belonging to a fundamental region of the symmetry group D_n (nm). In both cases, the rotation and dilatation center must coincide. Existing models in nature with similarity symmetry groups of the type D_nK (nmK) are the cause of the frequent occurrence of corresponding similarity symmetry rosettes in ornamental art (Figure 3.4). A fundamental region of the symmetry group D_nK (nmK) is the section of the fundamental regions of the symmetry groups D_n (nm) and K with the same invariant point. Due to the existence of at least one reflection in every symmetry group of the type D_nK (nmK), there is no enantiomorphism. Polar radial rays exist.

The simplest symmetry group of the type C_nL (nL) is the symmetry group L (n=1) generated by the dilative rotation L = L(k,q) - a composite transformation representing the commutative product of a dilatation K and the rotation S with the rotation angle q. Under the action of the transformation L, every vector (A,B) defined by two original points A, B, is transformed onto the vector (A',B' of the intensity |kAB|, defined by the image points A' = L(A), B' = L(B), which forms with the vector (A,B) the oriented angle q (for k > 0) or p-q (for k < 0). The following vector equalities: |(A',B')| = |k|(A,B)|, (A,B) °(A',B')/kAB² = cosq hold. Due to the maintenance of the angle between two original vectors, all the angles and the form of an original figure remain unchanged under the action the transformation L. Hence, the equiangularity and equiformity are the properties of a dilative rotation. Where the angle q of the dilative rotation L is a rational one ( q = pp/q, (p,q) = 1, p,q Î Z), among the symmetry transformations of the symmetry group L, there will be the dilatation K((-1)^pk^q). In a visual sense, it makes possible a division of the figure with the symmetry group L into the sectors of the dilatation K((-1)^pk^q) (A.V. Shubnikov, 1960) resulting in the appearance of polar radial rays. A sector of dilatation is any sector between two successive radial rays. A fundamental region of the symmetry group L is a part of the plane defined by two homologous lines of the transformation L.

There exist several ways of constructing figures with the similarity symmetry group L. Like the constructions of similarity symmetry groups of the type C_nK (nK), they can be divided into two basic kinds: constructions by using or not the metric property of a dilatation, which is a constituent part of the composite transformation L. Combinations of these methods are also possible. In ornamental art, the most frequent are non-metric constructions. The easiest method for construction of visual interpretations of the symmetry group L is the non-metric construction of a series of linked homologous asymmetric figures of the dilatation K(|k|), and afterward the rotation of every n-th figure (n Î N) through the angle nq (for k > 0) or n(p- q) (for k < 0). The metric construction is based on the construction of a series of homologous points of the dilative rotation L, which satisfy, simultaneously, both the rotation and the geometric progression of their distances from the invariant point. Besides the construction methods mentioned, for obtaining examples of the symmetry group L, we can use a desymmetrization of the continuous visually presentable similarity symmetry group D_Ą K ( ĄmK), a visual interpretation of which consists of a series of concentric circles satisfying the dilatation K (Figure 3.5a). A construction of a series of consecutive q-segments (for k > 0) or (p-q)-segments (for k < 0) followed by the elimination of reflections and maintenance of the equiformity of homologous asymmetric figures of the dilatative rotation L, which belong to the consecutive segments and consecutive circular rings, is very efficient for obtaining visual interpretations of the symmetry group L. Such a construction, in a certain sense, remains in some technical procedures (e.g., weaving baskets, etc.), but, because of the technical characteristics of the work itself, there usually occur deviations from the regularity dictated by the dilatation, the use of the simplest metric regularity - equidistance, and the disturbance of the similarity symmetry (Figure 3.6).

Figure 3.5

Visual interpretations of the continuous similarity symmetry groups: (a) DĄ K (ĄmK); (b) L1; (c) C2L1 (2L1).

Figure 3.6

Woven baskets of the American Indians, which suggest the similarity symmetry of rosettes C5L (5L) and C4L (4L).

Since every symmetry group L(k,q) for k < 0 can be reduced to the symmetry group L(|k|,p- q), a particular analysis of the symmetry groups L, depending on the sign of the coefficient k, is not necessary. Nevertheless, in ornamental art, examples of the symmetry groups L with an acute minimal angle of the dilative rotation L and k > 0, are more frequent. In the symmetry group L, there is the possibility of the enantiomorphism. If the dilative rotation angle is a rational one, i.e. q = pp/q, (p,q) = 1, p,q Î Z, there exist polar radial rays.

In nature, there are frequent and various examples of spiral forms. Most of them are the results of different rotational motions. The symmetry group L occurs as the symmetry group of complete natural forms or their parts, in non-living natural forms (e.g., as galaxies, whirlpools at turbulent motion of fluids, etc.) as well in at living creatures (the spiral tendency of shell growth in some mollusks, the spiral tendency of growth in certain plants or plant products, etc.).

As a fundamental geometric figure, and by using models in nature, the spiral became one of the most frequent dynamic symbols in the whole of art. Regarding its visual-symbolic meaning and its frequency of occurrence in ornamental art, it can be considered as the dynamic equivalent of the circle. The oldest examples of spirals date to the Paleolithic (Figure 3.7). In the further development of ornamental art, the spiral appears, most probably independently, in all cultures, distant both in place and time, as one of the basic ornamental motifs (J. Purce, 1975).

All the orbit points L(P) of a point P in general position with respect to the symmetry group L belong to a logarithmic, equiangular spiral. A logarithmic spiral is the orbit of a point of the plane E²\{O} , with respect to the continuous visually presentable conformal symmetry group L₁Z_I. A logarithmic spiral satisfies the condition of "uniform motion", according to H. Weyl (1952). It is the only plane curve with the property of equiangularity. This means it intersects all its radius-vectors under a constant angle. When the angle between a radius-vector and the corresponding tangent line is 90^° , the logarithmic spiral is reduced to a circle. The fact that every linear transformation of the plane transforms a logarithmic spiral onto the logarithmic spiral congruent to it, led J. Bernulli to name it "spira mirabilis" (A.A. Savelov, 1960). From the point of view of the theory of similarity symmetry, of special interest is the invariance of a logarithmic spiral with respect to certain similarity transformations. In a visual sense, Weyl's condition of the "uniform motion" is expressed by the fact that by the uniform rotation of a logarithmic spiral around its center, it is possible to realize the visual impression of the change in dimensions of the logarithmic spiral - its increase or decrease. This visual phenomenon shows the equivalence of the action of a dilatation and rotation with the same center. This property is used in applied art to decorate rotating elements (e.g., wheels) (Figure 3.8).

A bipolar, non-oriented logarithmic spiral corresponds to the visually presentable continuous conformal symmetry group L₁Z_I. An oriented logarithmic spiral corresponds to the visually presentable continuous similarity symmetry group L₁ (Figure 3.5b), the subgroup of the index 2 of the symmetry group L₁Z_I. An equiangular, logarithmic spiral is the invariant figure of this continuous conformal symmetry group and of its subgroups. By using that property, constructions based on the maintenance of the equiangularity and equiformity may be simplified. So that, it can serve as a basis for the construction of all similarity symmetry rosettes with the symmetry group L, by applying the desymmetrization method to the symmetry group L₁Z_I with the same dilative rotation angle (A.V. Shubnikov, 1960).

In nature, there are forms that almost perfectly satisfy the regularity of a logarithmic, equiangular spiral. This is, so called, the spiral tendency of growth. Despite the frequent occurrence of spiral forms in ornamental art, the subsequent use of similarity symmetry rosettes possessing the symmetry group L, was relatively seldom. Since the construction of a certain logarithmic, equiangular spiral is considerably more complicated than the construction of some other spiral (e.g., an Archimede's or equidistant spiral - a plane curve that can be constructed as the evolvent of a circle by a simple mechanical procedure), a logarithmic spiral is often replaced by an equidistant spiral. That results in a disturbance of the equiangularity and equiformity, and consequently, of the similarity symmetry.

The basic visual-symbolic characteristic of the symmetry group L is a double visual dynamism, caused by the visual suggestion of a rotational motion and centrifugal expansion, resulting from the rotational and dilatational component. Polar radial rays exist under the condition that q = pp/q, (p,q) = 1, p,q Î Z. A degree of the visual dynamism produced by corresponding similarity symmetry rosettes depends on the coefficient k and on the dilative rotation angle q. Changes in these parameters result in different visual impressions.

The symmetry group L is applied in painting works having the central perspective as the element, or even as a basis of the complete central dynamic composition of the work (e.g., in the baroque, in Tintoretto's works), creating thus the visual impression of an expanding rotational motion.

Similarity symmetry groups the type C_nL (nL) are formed by composing the symmetry groups L and C_n (n) with the same invariant point. Corresponding similarity symmetry groups can be constructed multiplying by the dilative rotation L a figure with the symmetry group C_n (n), belonging to a fundamental region of the symmetry group L, or multiplying by the n-fold rotation a figure with the symmetry group L, belonging to a fundamental region of the symmetry group C_n (n) (Figure 3.9a, 3.10). In both cases the rotation center and dilative rotation center coincide. Constructional methods used are analogous to that considered with the symmetry group L. In ornamental art, especially when the metric construction method is applied, deviations from the regularity dictated by the metric property of the dilative rotation L, frequently occur. A tendency toward maximal constructional simplicity results in the appearance of the equidistance, disturbance of the equiangularity and equiformity, desymmetrization of the similarity symmetry group C_nL (nL) and its reduction to the symmetry group C_n (n).

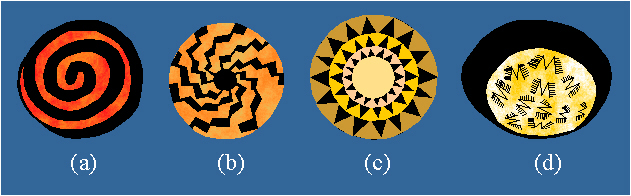
Figure 3.9

Examples of similarity symmetry rosettes in the art of Neolithic and pre-dynastic period of the ancient civilizations, around 4500-3500 B.C.: (a) Egypt; (b), (c) examples of rosettes with the similarity symmetry group of the type CnL (nL) and DnL (nmL), Egypt and Iran; (d) example of the rosette with the similarity symmetry group of the type DnL (nmL), Susa ceramics.

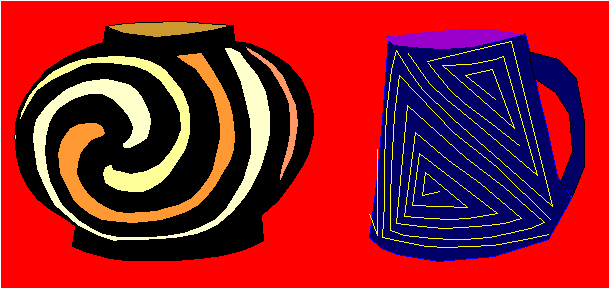
Figure 3.10

Examples of ornamental motifs in the ceramics of the American Indians, that suggest similarity symmetry.

By applying the criterion of maximal symmetry, it is possible to eliminate certain repetitions and overlappings of symmetry groups, otherwise occurring within the type C_nL (nL). The existence of the n-fold rotation with the rotation angle 2p/n and the dilative rotation L with the dilative rotation angle q (for k > 0) or ( p-q) (for k < 0), within the symmetry group C_nL (nL) results in the appearance of the new dilative rotation L' with the minimal dilative rotation angle q', which is less than the dilative rotation angle q. According to the criterion of maximal symmetry, every symmetry group C_nL (nL) can be considered as the symmetry group C_nL' (nL'). If we accept the condition C_nL₀=C_nL(k,0) = C_nK, the type C_nK (nK) is the subtype of the type C_nL (nL). For n - an odd natural number and k < 0 , the relationship C_nL_2n=C_nL(k,p/n) = C_nK (|k|) = C_nK holds. For n - an odd natural number, according to the above relationship and the relationship C_nK=C_nL_2n, holding for n - an odd natural number and k < 0, we can conclude that the types C_nK (nK) and C_nL_2n (nL_2n), are dual with respect to the change of the sign of the coefficient k.

A fundamental region of the symmetry group C_nL (nL) is the section of the fundamental regions of its generating symmetry groups C_n (n) and L with the same invariant point. Between symmetry groups of the type C_nL (nL) there will be no essential difference depending on the sign of the coefficient k. Since examples of rosettes with the symmetry group C_n (n) are relatively rare in ornamental art, the same refers to similarity symmetry rosettes with the symmetry group C_nL (nL). Such similarity symmetry rosettes occur in enantiomorphic modifications. If the angle of the dilative rotation L is a rational one, polar radial rays exist. Then, the existence of a dilatation as the element of the symmetry group C_nL (nL) makes it possible to divide the corresponding similarity symmetry rosette into the sectors of the dilatation.

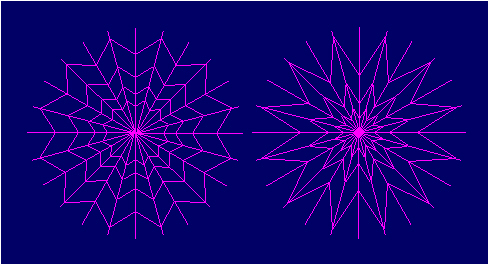
Similarity symmetry rosettes with the symmetry group C_nL (nL) (Figure 3.9b, 3.11c, e) possessing a very high degree of visual dynamism, caused by the polarity of both the relevant components - n-fold rotation and dilative rotation L - produce a visual impression of centrifugal rotational expansion. The existence of models in nature, the dynamic visual impression that suggest, their expressiveness and visual-symbolic function resulted in the appearance and use of similarity symmetry rosettes with the symmetry group C_nL (nL) in ornamental art.

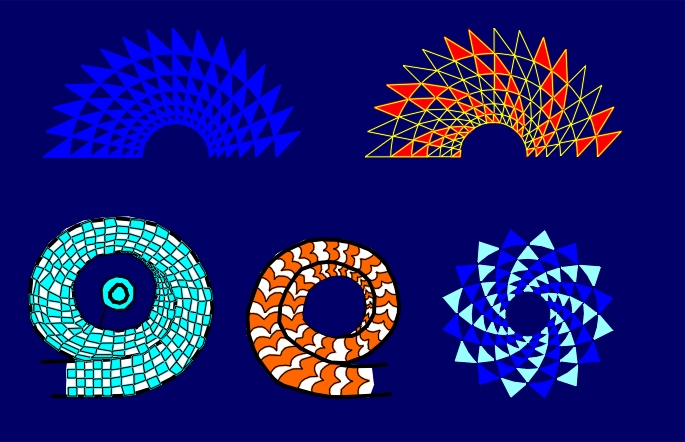
Figure 3.11

Examples of similarity symmetry rosettes in Greek and Byzantine ornamental art.

Similarity symmetry groups of the subtype C_nL_2n(k,p/n) (nL_2n(k,p/n)) can be derived by desymmetrizations of similarity symmetry groups of the type D_nL (nmL), which are more frequent in ornamental art. By choosing an appropriate desymmetrization and eliminating reflections of the symmetry group D_nL ( nmL), the symmetry group C_nL_2n (nL_2n) can be obtained. By the antisymmetry desymmetrization of the symmetry group D_nL (nmL ), the antisymmetry group D_nL/C_nL_2n (nmL/nL_2n), treated by the classical theory of symmetry as the symmetry group C_nL_2n (nL_2n) belonging to the type C_nL (nL), can be derived (Figure 3.12b).

Figure 3.12

Examples of similarity symmetry rosettes in Roman ornaments.

Every symmetry group of the type D_nL (nmL) is the composition of the symmetry groups L(k,p/n) and D_n ( nm) with the same invariant point. A fundamental region of the symmetry group D_nL (nmL) is the section of fundamental regions of these two symmetry groups. Similarity symmetry rosettes with the symmetry group D_nL (nmL) (Figure 3.9c, d, 3.12c, 3.13) can be constructed multiplying by the dilative rotation L a rosette with the symmetry group D_n (nm), belonging to a fundamental region of the symmetry group L, where the rosette center and the dilative rotation center coincide. Construction methods used for obtaining similarity symmetry rosettes with the symmetry group D_nL (nmL) are analogous to the construction methods previously discussed, used with similarity symmetry groups of the type C_nL ( nL). Owing to a very high degree of symmetry, the existence of models in nature (e.g., flowers and the fruits of certain plants) and frequent applications of the symmetry group of rosettes D_n (nm), the type D_nL (nmL), regarded from the point of view of ornamental art, is one of the largest and most heterogeneous types of the similarity symmetry groups of rosettes S₂₀. For n - an even natural number, there is no difference between individual symmetry groups of the type D_nL (nmL), depending on the sign of the dilatation coefficient k, but for n - an odd natural number and k < 0, the relationship D_nL=D_nL(k,p/n) = D_nK(|k|) = D_nK holds. According to this relationship and the relationship D_nK=D_nL, holding for n - an odd natural number and k < 0, the types D_nK (nmK) and D_nL (nmL) are dual with respect to the change of the sign of the coefficient k. Owing to a rational angle of dilative rotation L, q = p/n, there are the polar radial rays - the axes of the dilatation K(k²), incident to the reflection lines. Therefore, it is possible to divide a similarity symmetry rosette with the symmetry group D_nL (nmL) into the sectors of the dilatation. Enantiomorphic modifications do not exist.

Figure 3.13

Examples of Roman floor mosaics with the similarity symmetry groups of the type DnL (nmL).

A similarity symmetry rosette with the symmetry group D_nL (nmL) can be simply derived from a similarity symmetry rosette with the symmetry group D_nK (nmK) by its "centering" - by rotating every second set of its fundamental regions, homologous regarding transformations of its subgroup D_n (nm), through the angle q = p/n. The symmetry group D_nL (nmL) can be derived also by a desymmetrization of the symmetry group D_nK (nmK). Since the symmetry group D_nL (nmL) is the subgroup of the index 2 of the symmetry group D_nK (nmK), by using the antisymmetry desymmetrization, the antisymmetry group D_nK/ D_nL (nmK/nmL), treated by the classical theory of symmetry as the symmetry group D_nL (nmL), can be obtained.

Similarity symmetry rosettes with the symmetry group D_nL (nmL) possess a specific unity of visual dynamism and stationariness, produced, respectively, by the dynamic component - dilative rotation L - and by the static component - subgroup D_n (nm). The reflections of this subgroup cause the non-polarity of rotations and alleviate the dynamic visual effect produced by the dilative rotation L, which suggests an impression of centrifugal expansion. Changes of the shape of a fundamental region, which influences the visual impression, in similarity symmetry groups of the type D_nL (nmL) are restricted to the possible use of curvilinear boundaries, which do not belong to reflection lines. The other boundaries must be rectilinear. In all the similarity symmetry groups containing dilative rotations, a degree of visual dynamism or stationariness can vary according to the choice of parameters k, q... The spectrum of possibilities includes different varieties. This range from visually dynamic similarity symmetry rosettes with the symmetry group C_nL ( nL), with an irrational angle of the dilative rotation L, to similarity symmetry rosettes with a rational angle, which offer a perception of the sectors of dilatation, through to static similarity symmetry rosettes with the symmetry group D_nL (nmL), with the coefficient of dilative rotation k » 1, which are, by their visual properties, similar to rosettes with the symmetry group D_n (nm).

The simplest among similarity symmetry groups of the type C_nM (nM) (Figure 3.14, 3.15) is the symmetry group M (n = 1) generated by the dilative reflection M - a composite transformation representing the commutative product of a dilatation and reflection. A fundamental region of the symmetry group M is a part of the plane defined by two homologous lines of the dilative reflection M. The polar radial rays exist. Due to the presence of the indirect transformation - dilative reflection M - there is no the possibility of the enantiomorphism.

Figure 3.14

The rosette with the similarity symmetry group C4M (4M) in the ornamental art of Oceania, Bali.

Figure 3.15

Examples of similarity symmetry rosettes in the ornamental art of Oceania (New Zealand, New Guinea, Solomon Islands).

There are several ways to construct figures with the similarity symmetry group M. They can be divided into non-metric constructions, based on the use of the non-metric properties of the dilatation K - parallelism or antiparallelism of homologous vectors of the dilatation K, equiformity, equiangularity and linking of its successive homologous asymmetric figures - and metric constructions, based on the use of the metric property of the dilatation K that is a constitutive part of the composite transformation M(k,m). Such a construction always begins with the metric construction of a series of homologous asymmetric figures of the dilatation K. After that, it is necessary to copy by the reflection in the reflection line m, every second homologous figure mentioned. Combinations of these methods are also possible.

For the needs of ornamental art, probably the most efficient is the non-metric construction, consisting of the construction of a series of asymmetric figures that satisfy the dilatation K(|k|), by applying the linking of successive homologous asymmetric figures of the dilatation K. After that, every second figure must be copied by the reflection with the reflection line m for k > 0, or by the reflection with the reflection line m' perpendicular to the reflection line m in the invariant point for k < 0. In line with this, when analyzing the similarity symmetry group M, it is not necessary to discern the cases of k > 0, k < 0.

By applying the metric construction method, aiming for maximal constructional simplicity, there frequently occur deviations from the requirements of similarity symmetry. In such a case, the geometric progression mentioned above, is replaced by an analogous arithmetic progression.

Since a dilative reflection is present in nature (e.g., in the arrangement and growth of leaves in certain plants), natural models are imitated by ornamental art. Therefore, the similarity symmetry group M appears even in Paleolithic ornamental art, although followed by deviations with respect to the geometric consistency. The other reason for the origin and the use of the similarity symmetry group M can be found in the visual effect and symbolic meanings which corresponding similarity symmetry rosettes possess. Owing to the polarity of the radial ray incident to the reflection line m and due to the dynamic visual properties of the dilative reflection, similar to that of a glide reflection, figures with the symmetry group M can serve as the visual symbols of oriented, polar alternating phenomena of a growing intensity. It is, probably, the origin and reason for the frequent occurrence of similarity symmetry rosettes with the symmetry group M in primitive art. They occurr independently, or within more complex similarity symmetry rosettes with a symmetry group of the type C_nM (nM) (Figure 3.14, 3.15). By varying the dilatation coefficient k and the angle between the reflection line m and the radial ray of the dilatation K(k²) which belongs to the symmetry group M and generates its subgroup of the index 2, it is possible to emphasize or alleviate the dynamic visual effect produced by the polar radial ray, which goes from suggesting an impression of dynamism, similar to that produced by a glide reflection, to an impression of stationariness similar to that produced by a reflection.

The use of the similarity symmetry group M in painting, comes to its full expression when presenting objects with the symmetry group 1g by applying the central perspective.

Similar characteristics of all the similarity symmetry groups of the type C_nM (nM) are conditioned by the essential properties of the similarity symmetry group M. Similarity symmetry groups of the type C_nM (nM) are the result obtained when composing the symmetry groups M and C_n (n) with the same invariant point. Similarity symmetry rosettes with the symmetry group C_nM ( nM) can be constructed by multiplying by the n-fold rotation a figure with the similarity symmetry group M, belonging to a fundamental region of the symmetry group C_n (n), or multiplying by the dilative reflection M a figure with the symmetry group C_n (n), belonging to a fundamental region of the symmetry group C_n (n). In both cases the rosette center and the dilative reflection center must coincide. The application of the non-metric construction method, combined with the use of the linking of asymmetric homologous figures of the dilative reflection M, is also possible. With the use of the metric construction method there often occur deviations from the regularities of the similarity symmetry group M - the replacement of the geometric progression mentioned above with a corresponding arithmetic progression, the disturbance of equiformity and equiangularity, and, consequently, of the similarity symmetry. These deviations are the result aiming for maximal constructional simplicity.

A fundamental region of the symmetry group C_nM (nM) is the section of fundamental regions of the symmetry groups C_n ( n) and M with the same invariant point. Within the type C_nM (nM), there will be no essential differences between individual symmetry groups, caused by the sign of the dilatation coefficient k. Every symmetry group C_nM(k,m), for n - an odd natural number and k < 0, can be treated as the symmetry group C_nM(|k|, m'), where by m' is denoted the reflection line perpendicular in the invariant point of the dilative reflection M(k,m) to the reflection line m. Hence, for n - an odd natural number and k < 0, the relationship C_nM(k,m)=C_nM(k,m') holds. Similarity symmetry rosettes with the symmetry groups C_nM(k,m) and C_nM(-k,m) will differ between themselves regarding the position of the dilative reflection axis, only for n - an odd natural number, while for n - an even natural number, there will be no such difference. There are no enantiomorphic modifications.

Reasons for the appearance and the use of similarity symmetry rosettes with the symmetry group C_nM (nM) in ornamental art, can be found in the imitation of natural forms, in certain arrangements of leaves and in the growth of some plants, combined with a decorative effect of rosettes with the symmetry group C_n (n). Among all similarity symmetry rosettes, those with similarity symmetry groups of the type C_nM (nM) possess the maximal degree of visual dynamism, conditioned by two dynamic components - the n-fold rotation and dilative reflection M, which combines by itself the visual dynamism of alternating motion and that of centrifugal expansion, caused by its dilative component. The intensity of the dynamic visual effect can be influenced by choosing the parameter k and the position of the reflection line m.

The symmetry groups C_n (n) and M are relatively rare in ornamental art. The same refers to the similarity symmetry groups of the type C_nM (nM), formed as their compositions. A similarity symmetry group C_nM (nM) can be obtained also by a desymmetrization of the symmetry group D_nK (nmK) or D_nL (nmL), examples of which are, due to their higher degree of symmetry, visual and constructional simplicity, more frequent in ornamental art.

Desymmetrizations achieved by a dichromatic coloring often result in antisymmetry groups of the type D_nK/C_nM ( nmK/nM) or D_nL/C_nM (nmL/nM), which in the classical theory of symmetry are considered within the type C_nM (nM). The same can be realized by suitable classical-symmetry desymmetrizations.

Similarity symmetry groups of the type D_nM (nmM), the existence of which was proposed by A.V. Shubnikov (1960), coincide to the similarity symmetry groups of the type D_nL (nm).

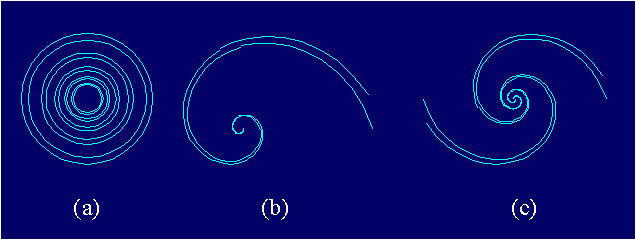
Among the continuous similarity symmetry groups of rosettes S₂₀, the symmetry groups D_Ą K (ĄmK) and C_nL₁ (nL₁) will have adequate visual interpretations, without using textures (Figure 3.5). As a visual model of the symmetry group D_Ą K (ĄmK), a series of concentric circles can be used - this model being obtained multiplying by the dilatation K two different concentric circles with the center incident with the dilatation center.

Adequate visual interpretations of all the other continuous similarity symmetry groups can be obtained only by using textures - the average even density of some elementary asymmetric figure, arranged according to the given continuous symmetry group. Concerning physical interpretations, all the continuous similarity symmetry groups have adequate interpretations, which can be realized by using physical factors (e.g., motion, rotation, the effect of a physical field, etc.).

The central problem with the similarity symmetry groups of rosettes S₂₀ and their examples in ornamental art is the question of the construction of corresponding similarity symmetry rosettes. As basic construction methods, it is possible to distinguish, first, the non-metric method, based, directly or indirectly, on the parallelism of homologous asymmetric figures of a dilatation, their equiangularity and equiformity; second, the metric method, founded on the fact that the distances of homologous points of the similarity transformations K, L, M from the invariant point form a geometric progression; and, third, combinations of these methods.

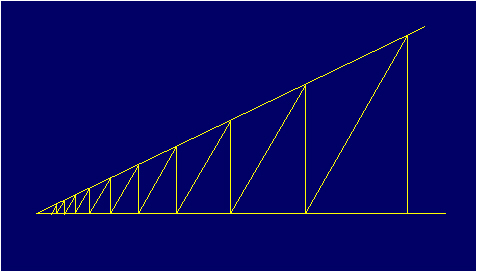
The non-metric construction method gives the best results, guaranteeing that equiformity and equiangularity will be respected, and may serve for the direct construction of similarity symmetry rosettes of the type C_nK (nK) or D_nK (nmK) with the dilatation coefficient k > 0, by applying the linking of successive homologous asymmetric figures of the dilatation K or dilative reflection M (Figure 3.3, according to A.V. Shubnikov, 1960). In the other cases, when similarity symmetry transformations are composite transformations, this means, in symmetry groups of the types C_nK (nK), D_nK (nmK) with k < 0, and all the symmetry groups of the types C_nL (nL), D_nL (nmL), C_nM (nM), it is not possible to use exclusively linking and parallelism. In these cases, after the first part of the construction, the copying of successive homologous asymmetric figures of the dilatation K (k > 0) by means of corresponding rotations and reflections becomes indispensable, so the construction becomes more complicated. In those cases where is not possible to link homologous asymmetric figures of a dilatation, because of the complexity of construction, the non-metric method has a relatively limited application.

The metric construction method shows its superiority, in the sense of constructional simplicity, in those situations when the non-metric construction method is difficult to apply - with composite similarity transformations or with unlinked homologous asymmetric figures obtained by dilatation. A negative aspect of the metric construction method, coming to its expression in ornamental art, is the possibility for replacing the geometric progression mentioned above by a corresponding arithmetic progression, aiming for maximal constructional simplicity. Such an inconsistent application of the metric construction method unavoidably disturbs the equiangularity, equiformity, and consequently, the similarity symmetry.

In ornamental art we can find many similarity symmetry rosettes, formed by the inconsistent use of the construction methods mentioned. Such rosettes do not satisfy the similarity symmetry but only suggest it. In early ornamental art, this is not the exception but the rule.

Both construction methods mentioned are used to construct similarity symmetry rosettes, formed by applying the similarity transformations K, L, M on a rosette with the symmetry group C_n (n) or D_n (nm) belonging to a fundamental region of the corresponding similarity symmetry group K, L or M. An opposite approach - the multiplication of a figure with the similarity symmetry group K, L, M, by the symmetries of the symmetry group C_n (n) or D_n (nm) - is not so frequent. Such a construction requires a better understanding of the similarity symmetry, especially concerning the fundamental regions of the generating groups, to avoid the possible overlapping of figures. In all those cases, the generating symmetry group of rosettes and the similarity symmetry group possess the same invariant point.

The desymmetrization method is not an independent construction method. It can be used exclusively if we know similarity symmetry groups of rosettes with a higher degree of symmetry, which can be reduced to a lower degree of symmetry by the elimination of certain symmetry elements, to derive their similarity symmetry subgroups. However, since similarity symmetry groups of a higher degree of symmetry, due to the principle of visual entropy, are more frequent and much older, this construction method has been abundantly used in ornamental art, with the classical-symmetry, antisymmetry and color-symmetry desymmetrizations.

Like the antisymmetry and colored symmetry groups of rosettes, friezes and ornaments, such desymmetrizations of similarity symmetry groups are of a somewhat later date, appearing in ornamental art with dichromatic and polychromatic ceramics (in the Neolithic and in the period of the ancient civilizations). Classical-symmetry desymmetrizations can be used to derive similarity symmetry subgroups of the arbitrary index of the given similarity symmetry group. Desymmetrizations of the continuous visually presentable similarity symmetry groups of the type D _ĄK (ĄmK), which can be visually interpreted by a system of concentric circles, obtained from two different concentric circles multiplied by the dilatation K with the same center, frequently occur (Figure 3.5a). This continuous similarity symmetry group is a perfect basis on which to apply the desymmetrization method. Continuous similarity symmetry groups of the type C_nL₁ (nL₁) (Figure 3.5b, c) are based on the continuous visually presentable conformal symmetry group L₁Z_I, which can be visually interpreted by the corresponding logarithmic spiral. Therefore, they make possible a very simple transition from the visually presentable continuous, to the corresponding discrete similarity symmetry groups.

The classical-symmetry desymmetrization method can be very successfully applied on the similarity symmetry groups generated by the symmetry group D_n (nm), to obtain their subgroups, generated by the symmetry group C_n (n). Since the symmetry group C_n (n) is the subgroup of the index 2 of the symmetry group D_n (nm), there is a possibility for antisymmetry desymmetrizations.

More detailed information on possible desymmetrizations of similarity symmetry groups can be found in the table of the group-subgroup relations existing between different types of the similarity symmetry groups of rosettes, and in the tables of antisymmetry and color-symmetry desymmetrizations.

Since the continuous similarity symmetry groups D_ĄK (ĄmK) and C_nL₁ ( nL₁) are visually presentable, very important are the group-subgroup relations between the continuous and discrete similarity symmetry groups of rosettes S₂₀: D_ĄK ® DK, DL, CL₁ ® CL. Between the different types of discrete similarity symmetry groups, the following relations hold: DL ® DK ® CM ® CK, using the symbols D, C instead of the symbols D_n, C_n, for denoting the group-subgroup relations between the types, and not between the individual symmetry groups.

When establishing the group-subgroup relations between the individual similarity symmetry groups of rosettes S₂₀ and their subgroups, we can use the group-subgroup relations existing between the symmetry groups C_n (n), D_n (nm) and the group-subgroup relations between the symmetry groups K, L, M, since all the similarity symmetry groups of rosettes S₂₀ are derived as the superpositions of the symmetry groups mentioned, i.e. as the extensions of the symmetry groups of rosettes G₂₀: C_n (n), D_n (nm) by the similarity transformations K, L, M. For the discrete similarity symmetry groups K, L, M, the following relationships hold: [K:K(k^m)] = m, [M: M(k^m)] = m, [L:L(k^m,kq)] = m (m Î N). For a rational angle of the dilative rotation q = pp/q, (p,q) = 1, p,q Î Z, the following relationships hold: [L:L(k^q,qq)] = q and L (k^q,qq) = L((-1)^pk^q,0) = K((-1)^pk^q), showing that every symmetry group L with a rational angle of dilative rotation q contains the subgroup generated by the dilatation K((-1)^pk^q). The relationship [M:K(k²)] = 2 highlights the existence of the subgroup of the index 2 generated by the dilatation K(k²) in every symmetry group M, while the relationship C_nK=C_nL(k,0) = C_nL₀ highlights the different type possibilities for the symmetry groups C_nK (nK). This means that they can be discussed within the type C_nL (nL), as the subtype C_nL₀ (nL₀).

By accepting the criterion of subordination, by treating the symmetry group K within the type C_nL (in accordance with the relationship K=L₀), and the type C_nK (nK) as the subtype of the type C_nL (nL) (in accordance with the relationship C_nK=C_nL₀), the whole discussion on the discrete similarity symmetry groups of rosettes can be reduced to the analysis of the symmetry groups of the types C_nL (nL), C_nM ( nM), D_nK (nmK) and D_nL (nmL). The criterion of the maximal symmetry can be introduced even between individual symmetry groups of the type C_nL (nL), where the symmetrization caused by a superposition of the n-fold rotation and the rotational component of the dilative rotation L results in the change of the minimal angle of the dilative rotation, and in the appearance of the new dilative rotation L', i.e. in the new symmetry group C_nL' (nL').

In the table of antisymmetry desymmetrizations of discrete similarity symmetry groups of rosettes, the symbols of antisymmetry groups, i.e. the corresponding antisymmetry desymmetrizations, are given in the group/subgroup notation G/H. The symbol q' corresponds to a newly derived minimal angle of the dilative rotation L'.

The table of antisymmetry desymmetrizations of similarity symmetry groups of rosettes S₂₀:

Besides the possibilities to apply the antisymmetry desymmetrization method, this table gives evidence for all the subgroups of the index 2 of any given discrete similarity symmetry group of rosettes. By using data given by A.M. Zamorzaev (1976), it is possible to compare similarity antisymmetry groups with the corresponding crystallographic antisymmetry groups of polar, oriented rods G₃₁. A complete catalogue of the similarity antisymmetry groups of rosettes S₂₀' is given by S.V. Jablan (1986b).

The color-symmetry desymmetrizations of the discrete crystallographic similarity symmetry groups of rosettes can be partially considered by using the work of E.I. Galyarski (1970, 1974b), A.M. Zamorzaev, E.I. Galyarski, A.F. Palistrant (1978), and A.F. Palistrant (1980c).

Different problems of tiling theory (B. Grünbaum, G.C. Shephard, 1987) are extended to the similarity symmetry groups of rosettes S₂₀ by E.A. Zamorzaeva (1979, 1984). In the works mentioned, a link is established between the similarity symmetry groups of rosettes S₂₀, the symmetry groups of polar oriented rods G₃₁ and corresponding symmetry groups of ornaments G₂, resulting in the following relationships: C_nK ( nK), C_nL (nL) @ p1, C_nM ( nM) @ pg, D_nK (nmK) @ pm, D_nL (nmL) @ cm. In this way, different problems of similarity symmetry plane tilings are reduced to the much better known problems of tilings that correspond to the symmetry groups of ornaments p1, pg, pm, cm. By using such an approach, the problems of isohedral and 2-homeohedral similarity symmetry plane tilings are solved by E.A. Zamorzaeva.

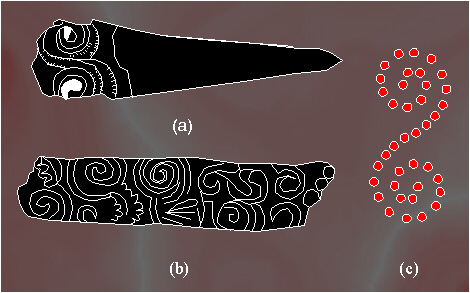
The chronology of similarity symmetry rosettes in ornamental art is connected with the problem of their construction. The oldest examples of rosettes suggesting similarity symmetry date to the Paleolithic and Neolithic, beginning with the appearance of the first spiral forms in art (Figure 3.7), series of concentric circles or concentric squares with parallel sides, and motifs based on natural models with the similarity symmetry group M or D₁K (mK), etc. In the Neolithic we come across more diverse and complex examples of rosettes with similarity symmetry groups of the type C_nL (nL) or D_nL (nmL) (Figure 3.9). Already in the Neolithic and in the ornamental art of ancient civilizations, there are examples of all the types of similarity symmetry groups of rosettes. Though, almost unavoidably, there are deviations from geometric regularity, these being due to the approximate constructions used in ornamental art. Ornamental motifs with the application of similarity symmetry reached their peak in the ornamental art of Rome and Byzantium (Figure 3.11-3.13), mainly in floor mosaics. Here we find examples of all the types of the similarity symmetry groups of rosettes, without any deviations from strict geometric regularity.

One of the conditions necessary for the appearance of corresponding similarity symmetry rosettes in ornamental art is the existence of models in nature, i.e. a spiral tendency in nature, expressed through the way of growth of certain living beings or as a result of rotational motions (e.g., whirlpools in a turbulent fluid motion, etc.). In the earlier periods of ornamental art, it is possible to note the imitation of models in nature that possess similarity symmetry. In the further development of ornamental art, a visual-symbolic component based on a suggestion of the impression of centrifugal expansion, produced by similarity symmetry rosettes, became the main reason for the use of similarity symmetry. After empirically solving the construction problems and discovering all the symmetry possibilities, i.e. all the types of the similarity symmetry groups of rosettes, primary symbolic meanings retreated into a concern for decorativeness. That opened new possibilities for the enrichment and variety of similarity symmetry rosettes in ornamental art.

Like with the symmetry groups of rosettes G₂₀, where rosettes with the symmetry groups of the type D_n (nm) are more frequent than rosettes with the symmetry groups of the type C_n (n), the principle of visual entropy and numerous models in nature caused the dominance of rosettes with similarity symmetry groups of the type D_nK (nmK), D_nL (nmL), over those with similarity symmetry groups of the types C_nK (nK), C_nM (nM), C_nL (nL). As generating symmetry groups of the type D_n ( nm), most frequently are used symmetry groups of rosettes D₁ (m), D₂ (2m), D₄ (4m), D₆ (6m), etc., mainly with n - an even natural number. In such rosettes the incidence of reflection lines to the fundamental natural directions - vertical and horizontal line - is possible.

A fundamental region of similarity symmetry groups offers the variation and the use of curvilinear boundaries. Rectilinear must be only those parts of the boundaries of the fundamental region that coincide with reflection lines. By changing the form of a fundamental region we can influence the intensity of static or dynamic visual impression produced by the given similarity symmetry rosette and intensify desired visual impression. In all similarity symmetry rosettes, it is possible to realize the corresponding (unmarked) isohedral plane tilings.

A basic visual property of similarity symmetry rosettes is the impression of centrifugal expansion, which these rosettes render to the observer. The intensity of that impression will depend primarily on the value of the coefficient k, on the form of a fundamental region or an elementary asymmetric figure belonging to the fundamental region, where the adequate use of acuteangular forms may stress a dynamic effect of a dilatation, occurring as the independent or dependent symmetry transformation. Polar, oriented rotations existing in subgroups of the type C_n (n) play the role of visual dynamic symmetry elements. Dilative reflections have a double, contradictory role, since they cause the absence of the enantiomorphism in groups of the type C_nM (nM). On the other hand, they increase visual dynamism, by suggesting the impression of a centrifugal alternating expansion. By varying the parameter k and the position of the reflection line m, we can stress the visual static or dynamic function of the dilative reflection M(k,m).

Enantiomorphic modifications do not exist in similarity symmetry groups of the types C_nM (nM), D_nK (nmK), D_nL (nmL), D_ĄK (ĄmK), i.e. in groups containing at least one indirect symmetry transformation. The presence of the dilatation K or K(k²) is obligatory in all the similarity symmetry groups of rosettes, except groups of the type C_nL (nL), which contain a dilatation only when the angle of the dilative rotation L is rational. Then is possible to perceive sectors of dilatation. Since the presence of a dilatation within the symmetry group C_nL (nL) increases the number of different symmetry transformations and simplifies the construction of corresponding rosettes, in line with the principle of visual entropy, similarity symmetry groups of the type C_nL ( nL), offering a division of the corresponding similarity symmetry rosettes into sectors of dilatation, will be more frequent in ornamental art than groups of the type C_nL (nL) with an irrational angle of the dilative rotation L.

Because of a high degree of symmetry and the possibility for the simple construction of their corresponding visual interpretations by desymmetrizations of groups of the type D_ĄK ( ĄmK), of special interest will be groups of the types D_nK (nmK) or D_nL (nmL). According to the principle of visual entropy, similarity symmetry groups generated by the symmetry groups of rosettes of the type D_n (nm), for n = 1,2,3,4,6,8,12,..., are the oldest and most frequent in ornamental art. In visual interpretations of the derived similarity symmetry groups of rosettes a dynamic visual component - the suggestion of a centrifugal expansion conditioned by dilatation - is in visual balance with the static component produced by reflections. The result is non-polarity of rotations and absence of the enantiomorphism. On the other hand, in the older ornamental art and that of primitive people, visually dynamic rosettes with similarity symmetry groups of the types C_nK (nK), C_nL (nL), C_nM (nM), C_nL₁ (nL₁), with polar rotations and dilative reflections, are very frequent. Their abundant use in ornamental art, is due to their symbolic function.

Besides serving as a basis for the application of the desymmetrization method, the tables of the group-subgroup relations between the types of similarity symmetry groups or between the individual groups are, at the same time, an indicator of symmetry substructures of a given similarity symmetry group. They represent the groundwork for the exact registering of the subentities mentioned, which with an empirical visual-perceptive approach is sometimes very difficult. The surveys given consist of a series of inclusion relations beginning with the maximal visually presentable continuous similarity symmetry groups of the types D_ĄK (ĄmK) and C_nL₁ (nL₁), including all discrete similarity symmetry groups and ending with the symmetry groups of rosettes D_n (nm) and C_n (n) and their subgroups. When discussing continuous similarity symmetry groups, only the visually presentable groups are considered, since ornamental art imposes this restriction. Visually non-presentable similarity symmetry groups will have their physical interpretations, owing to the possibility of including physical desymmetrization factors (e.g., a uniform rotation of a rosette with the similarity symmetry group D_ĄK (ĄmK) around the invariant point, when its symmetry group is reduced to the symmetry group C_Ą K (ĄK), or by using similar methods). In ornamental art, visual presentations of such continuous similarity symmetry groups can be obtained by using textures. As physical interpretations of these groups, we may consider different similarity symmetry structures realized by means of a physical field with a singular point, the intensity of which depends on distance from the singular point, according to the requirements of the similarity symmetry.

In analyzing the visual properties of similarity symmetry groups we can use, very efficiently, their visual interpretations: similarity symmetry rosettes, tables of the graphic symbols of symmetry elements and Cayley diagrams. Owing to the existence of the isomorphism between the similarity symmetry groups of rosettes S₂₀ and the symmetry groups of polar, oriented rods G₃₁, the properties of the similarity symmetry groups of rosettes S₂₀, the characteristics of similarity transformations and relations which are included in their presentations, will be, sometimes, more evident in the symmetry groups of polar, oriented rods G₃₁. The symmetry groups of rods G₃₁, that in the isomorphism mentioned correspond to the similarity symmetry groups of rosettes S₂₀, possess the same presentations and geometric characteristics. By analyzing the symmetry groups of rods G₃₁, the conclusion on the absence of the type D_nM (nmM) of the similarity symmetry groups of rosettes and its reduction to the type D_nL (nmL), becomes absolutely clear. The same is proved by the table of the symmetry groups of rods G₃₁ (A.V.Shubnikov, V.A.Koptsik, 1974) in which, because of the justification already given, there is no individual type ( a)(2n)_nã, consisting of groups isomorphic to similarity symmetry groups of the type D_nM (nmM) . These symmetry groups of rods are included in the type (a)(2n)_nm, consisting of groups isomorphic to the similarity symmetry groups of the type D_nL (nmL).

The problem of plane symmetry groups isomorphic to the symmetry groups of non-polar rods G₃₁ is solved in the theory of conformal symmetry introduced by A.M. Zamorzaev, E.I. Galyarski and A.F. Palistrant (1978), in the Euclidean plane with a singular point O removed, i.e. in the plane E²\{O} .

All the other problems in the field of visual interpretations of the similarity symmetry groups of rosettes S₂₀ - "objective" and "subjective" symmetry, problems of perceiving the objective symmetry and eliminating other visual symmetry factors, desymmetrizations or symmetrizations caused by physiological-physical reasons, the effect of the principle of visual entropy, problems of visual perception of substructures, treatment of symmetry groups of "real" similarity symmetry rosettes as finite factor groups of "ideal", infinite similarity symmetry groups of rosettes, etc. - can be discussed analogously to the similar problems of visual perception previously analyzed with the symmetry groups of rosettes G₂₀, friezes G₂₁ and ornaments G₂.

The chronological parallelism and the use of similar construction methods in ornamental art and the theory of similarity symmetry, the more profound connection between the similarity symmetry groups used in ornamental art and the theory of symmetry, the possibility of a different approach to ornaments treating them as models of geometric-algebraic structures and many other similar questions, are some of the problems raised in this work that demand a more detailed study.

NEXT

CONTENTS