| |
|
|
| |
Volume 4, Issue 4, Article 73 |
|
|
|
|
|
| |
A Variant of Jensen's Inequality
|
| |
|
Authors: |
A.McD. Mercer, |
|
| |
|
Keywords:
|
Jensen's inequality, Convex functions. |
|
| |
|
Date Received:
|
14/08/03 |
|
| |
|
Date Accepted:
|
27/08/03 |
|
| |
|
Subject Codes: |
26D15
|
|
| |
|
Editors: |
Peter S. Bullen, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
If 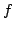 is a convex function the following variant of the classical Jensen's is a convex function the following variant of the classical Jensen's
Inequality is proved
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|



