Previous: Chapter 1: Prelude: Gauss mappings of plane curves
Up: Table of Contents
Next: Chapter 2: Examples 3a and 3b
Chapter 2
Gauss mappings of surfaces
For an embedding
X : U -> R3 of a
parameter domain U in R2 into Euclidean
3-space, the Gauss mapping
N : U-> S2 sends each point
(x, y) of U to the unit normal
N = (Xx x Xy) / |Xx x Xy|.
The Gauss mapping is singular precisely when
0 = Nx x Ny = K(x, y) Xx x Xy,
i.e. on the parabolic set where the Gaussian curvature
K(x, y) = 0.
In the terminology of Whitney [Wh] , the Gauss mapping N is
good if the gradient of K is never zero on the parabolic set.
If N is good, then the parabolic set is a smooth curve
(x(t), y(t)). The image
N(t) of this curve under the Gauss map is singular
precisely when N'(t) = 0. If N is
good, then N is excellent if N'(t) = 0
implies N"(t) 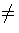 0. This ensures that the
singularities of the curve N(t) are cusps. Finally, if
N is excellent, then N is in general position if the
image of N(t) has no triple points or self-tangencies,
and no cusp point of N(t) coincides with another image
point of N(t).
0. This ensures that the
singularities of the curve N(t) are cusps. Finally, if
N is excellent, then N is in general position if the
image of N(t) has no triple points or self-tangencies,
and no cusp point of N(t) coincides with another image
point of N(t).
Whitney proved that a map of surfaces is excellent if and only if
its singularities are all equivalent (by smooth changes of
coordinates) to folds or cusps. Furthermore, a map of surfaces is
stable if and only if it is excellent and in general position. (For a
precise definition of stability and a discussion of Whitney's theorem,
see [A1] and [GolG].)
We begin our investigation of the singularities of the Gauss
mapping with a collection of key examples which exhibit all of the
geometric phenomena which we shall associate with these singularities.
Our first three examples are function graphs of the form
X(x, y) = (x, y, f(x, y)),
so the Gauss map is given by
N(x, y) = (-fx, -fy, 1)/[(1 + (fx) 2 + (fy)2]1/2
We can study the singularities of the Gauss mapping more easily in
this case by projecting centrally from the origin to the plane
z = 1 to get
(-fx, -fy, 1).
We then project to the xy-plane to get the composed mapping
Ñ(x,y) = (-fx, -fy)
Since the image of N is contained in the upper hemisphere,
and central projection is a diffeomorphism from the upper hemisphere
to the plane z = 1 , the modified Gauss
mapping Ñ will have the same singularities as N.
In particular N is singular precisely when the Jacobian matrix
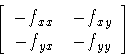
has rank less than two, i.e. when the discriminant
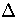 = (fxy)2 - fxx fyy
is zero.
= (fxy)2 - fxx fyy
is zero.
X(x,y) = (x, y, 1/3 x3 - 1/2 y2)
The modified Gauss mapping is
Ñ(x, y) = (-x2, y),
and the parabolic curve is obtained by solving
0 = 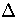 = (fxy)2 - fxx fyy = 2 x.
Since grad
= (fxy)2 - fxx fyy = 2 x.
Since grad 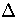 = (2, 0)
= (2, 0) 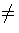 0, the
mapping Ñ is good. The parabolic curve can be parametrized
by
x(t) = 0, y(t) = t.
The modified Gauss mapping restricted to the parabolic curve is
Ñ(t) = (0, t), with
Ñ'(t) = (0, 1)
0, the
mapping Ñ is good. The parabolic curve can be parametrized
by
x(t) = 0, y(t) = t.
The modified Gauss mapping restricted to the parabolic curve is
Ñ(t) = (0, t), with
Ñ'(t) = (0, 1) 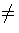 0, so
Ñ is excellent. Thus the Gauss map is stable, with a simple
fold along the parabolic curve.
0, so
Ñ is excellent. Thus the Gauss map is stable, with a simple
fold along the parabolic curve.
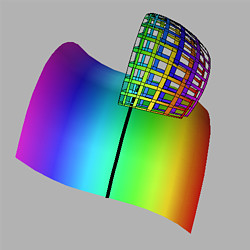
Figure 2.1
The shoe surface
and its spherical image.
X(x, y) = (x, y,  x4 + x2y - y2)
x4 + x2y - y2)
The modified Gauss mapping is then
Ñ(x, y) = (-4  x3 - 2 xy, -x2 + 2y)
and the parabolic curve is obtained by solving
0 =
x3 - 2 xy, -x2 + 2y)
and the parabolic curve is obtained by solving
0 = 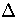 = (24
= (24  + 4) x2 + 4 y.
Since
grad
+ 4) x2 + 4 y.
Since
grad 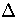 = ((48
= ((48  + 8) x, 4)
+ 8) x, 4) 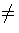 0,
the mapping Ñ is good for all
0,
the mapping Ñ is good for all  . The parabolic curve can
be parametrized by
x(t) = t, y(t) = -(6
. The parabolic curve can
be parametrized by
x(t) = t, y(t) = -(6  + 1) t2,
so Ñ restricted to the parabolic curve is
+ 1) t2,
so Ñ restricted to the parabolic curve is
Ñ(t) = (2(4  + 1) t3, -3 (4
+ 1) t3, -3 (4  + 1) t2)
+ 1) t2)
and Ñ'(t) = 0 implies
Ñ"(t) 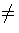 0 , if
0 , if 
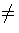 -1/4.
Therefore the Gauss map is stable if
-1/4.
Therefore the Gauss map is stable if 
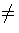 -1/4, with a
cusp at the origin. For
-1/4, with a
cusp at the origin. For  = -1/4, the entire parabolic
curve is sent to a single point by the Gauss map. (This is similar to
the situation which occurs at the top rim of a torus of revolution
-cf. examples 4 and 5 below.) If
= -1/4, the entire parabolic
curve is sent to a single point by the Gauss map. (This is similar to
the situation which occurs at the top rim of a torus of revolution
-cf. examples 4 and 5 below.) If  < -1/4,
f(x, y) =
< -1/4,
f(x, y) =  x4 + x2y - y2
has an absolute maximum at the origin. If
x4 + x2y - y2
has an absolute maximum at the origin. If  > -1/4,
f(x, y) has a topological saddle point at the
origin. The case
> -1/4,
f(x, y) has a topological saddle point at the
origin. The case  = 0 was first studied by Michael
Menn.
= 0 was first studied by Michael
Menn.
A related example of a surface with an unstable Gauss map is
X(x, y) = (x, y, 1/4 x4 - 1/2 y2)
The modified Gauss mapping is
Ñ(x,y) = (-x3, y)
and 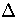 = 3 x2, so the
parabolic curve is the line x = 0. Since
grad
= 3 x2, so the
parabolic curve is the line x = 0. Since
grad 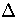 = 6x is zero on the parabolic curve,
the Gauss map is not good. Both this surface and Menn's surface occur
in the 3-parameter family
= 6x is zero on the parabolic curve,
the Gauss map is not good. Both this surface and Menn's surface occur
in the 3-parameter family
X(x, y) = (x, y, a x4 + b x2y + c y2)
also considered by Bleeker and Wilson [BlW, p. 286].
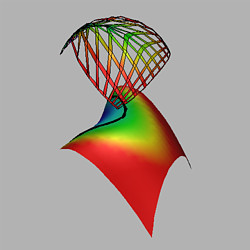
Figure 2.2
Menn's surface and its spherical image ( = -1/2).
= -1/2).
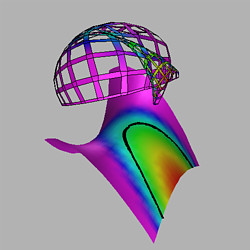
Figure 2.3
Menn's surface and its spherical
image ( = 1/4).
= 1/4).
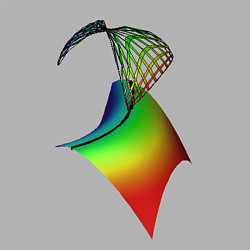
Figure 2.4
Menn's surface and its spherical
image ( = -1/4).
= -1/4).
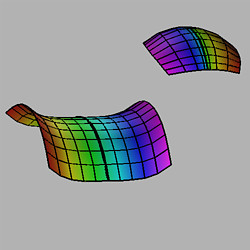
Figure 2.5
The surface
z = 1/4 x4 - 1/2 y2,
and its spherical image.
Previous: 1 Prelude: Gauss mappings of plane curves
Up: Table of Contents
Next: 2 Examples 3a and 3b
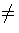 0. This ensures that the
singularities of the curve N(t) are cusps. Finally, if
N is excellent, then N is in general position if the
image of N(t) has no triple points or self-tangencies,
and no cusp point of N(t) coincides with another image
point of N(t).
0. This ensures that the
singularities of the curve N(t) are cusps. Finally, if
N is excellent, then N is in general position if the
image of N(t) has no triple points or self-tangencies,
and no cusp point of N(t) coincides with another image
point of N(t).
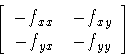
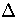 = (fxy)2 - fxx fyy
is zero.
= (fxy)2 - fxx fyy
is zero.

 x4 + x2y - y2)
x4 + x2y - y2)


